▎Achievements
A Riemannian Geometry Theory of Nonlinear Optical Properties of Materials
Bulk photovoltaic effect (BPVE) is a second-order nonlinear optical effect that generates dc photocurrents in a noncentrosymmetric solid under light irradiation. Topological semimetals emerge as efficient infrared and terahertz photodetectors due to this promising mechanism. Recently, Professor Guo of NTU’s Department of Physics, together with researchers from RIKEN and the University of Tokyo, Japan as well as Harvard University, USA, investigated the second-order optical conductivity in topological semimetals described by Dirac and Weyl models.(1) Through symmetry and power counting analysis, they showed that Dirac and Weyl points with tilted cones exhibit the leading low-frequency divergence. They also uncovered the complete quantum geometric meaning of the low-frequency BPVE (TABLE I). For example, they found that the injection current is controlled by the quantum metric and Berry curvature, whereas the shift current is governed by Christoffel symbols. They also performed material specific first-principles calculations, and discovered that gigantic photoconductivity at low frequencies is due to the divergent behavior of the geometric quantities near the Dirac and Weyl points. This work(1) brings new insights into the BPVE as well as the design of semimetal-based terahertz photodetectors.
The geometry of quantum states is a powerful concept for understanding the responses of electronic systems to static electromagnetic fields, as exemplified by the quantum Hall effect. However, it has been challenging to relate quantum geometry with optical responses. The main obstacle is that optical transitions are properties of a pair of states, while existing geometrical properties are defined for a single state. Professor Guo and his collaborators recently constructed a Riemannian geometry theory for all-order optical processes by identifying transition dipole moment matrix elements as tangent vectors (FIG. 1). They showed that optical responses can be generally thought of as manifestations of the Riemannian geometry of quantum states. They also applied their theory that third-order photovoltaic Hall effects are related to the Riemann curvature tensor, and demonstrated an experimentally accessible regime where they dominate the response (FIG. 1). Their intriguing discovery was recently published in the leading physics journal Nature Physics.(2)
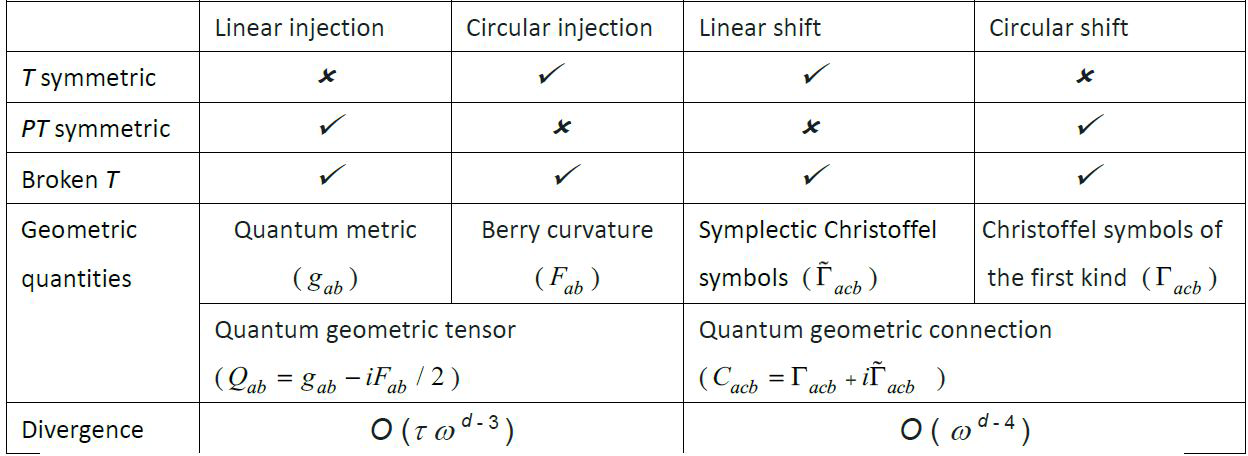
TABLE I: Low-frequency properties of dc photovoltaic responses: linear and circular injection currents as well as linear and circular shift currents, together with the corresponding quantum geometric quantities and leading divergence. T denotes time-reversal, ω is photon frequency, d denotes spatial dimensions, τ denotes photoelectron relaxation time.
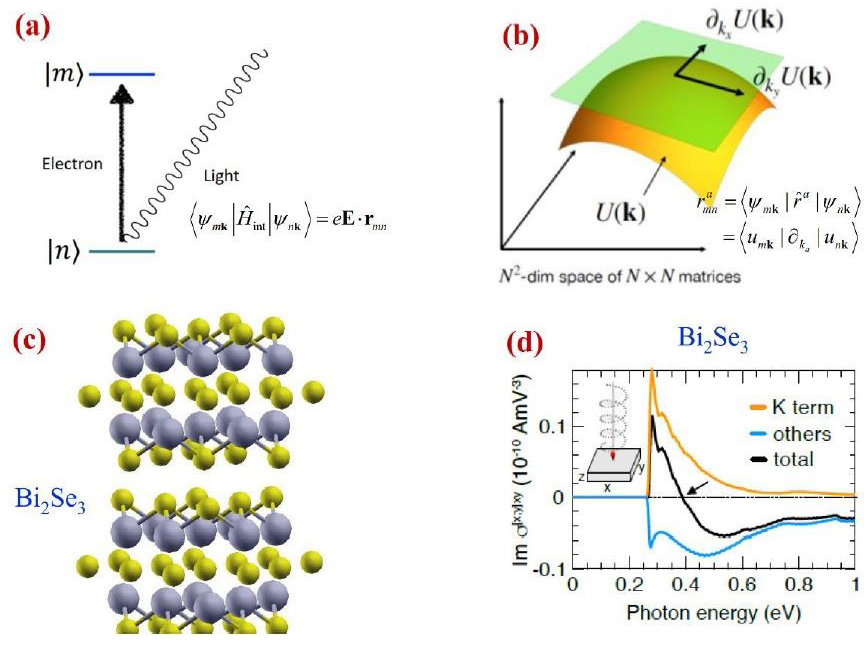
FIG. 1: (a) Optical transition and (b) geometry of the Bloch state: optical transition matrix elements as tangent basis vectors. (c) Structure of massive Dirac material Bi2Se3, and (d) calculated third-order circular photovoltaic Hall conductivity for Bi2Se3. As the theory predicts, the Hermitian curvature (K term) dominates the response near the band edge.


